STL-Setmap

前言

大家好，我是jiantaoyab，我们将进入到C++STL 的学习。STL在各各C++的头文件中，以源代码的形式出现，不仅要会用，还要了解底层的实现。源码之前，了无秘密。

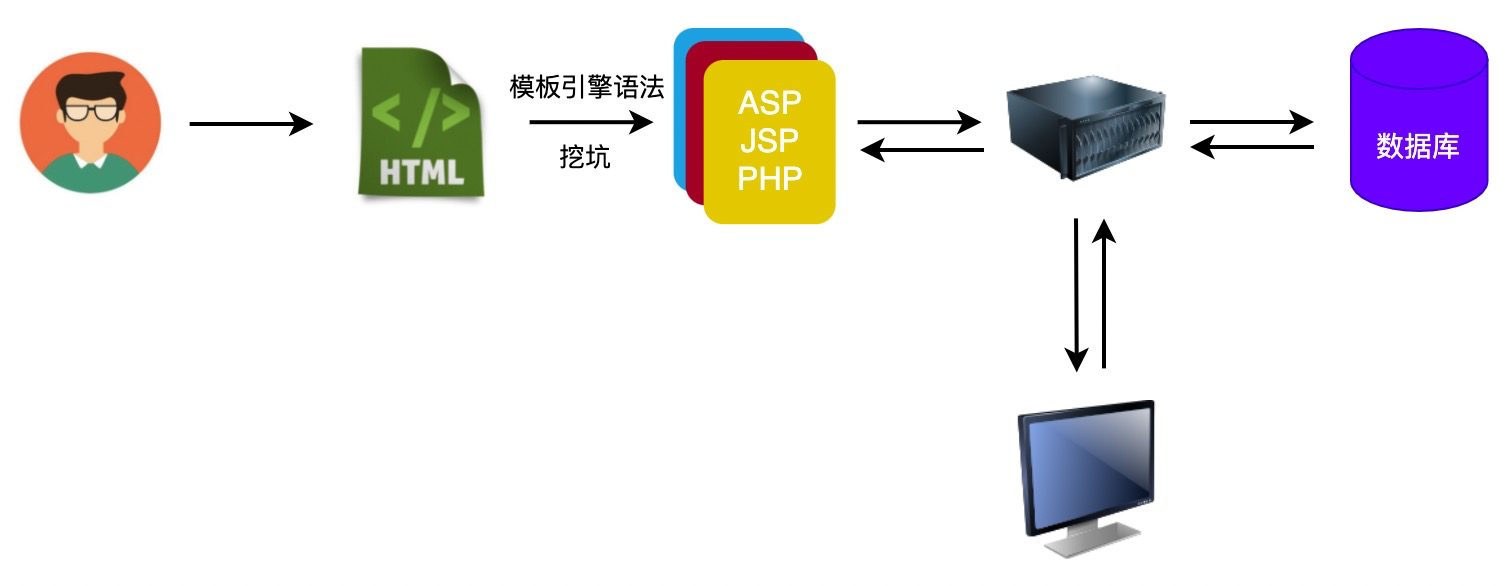
STL六大组件

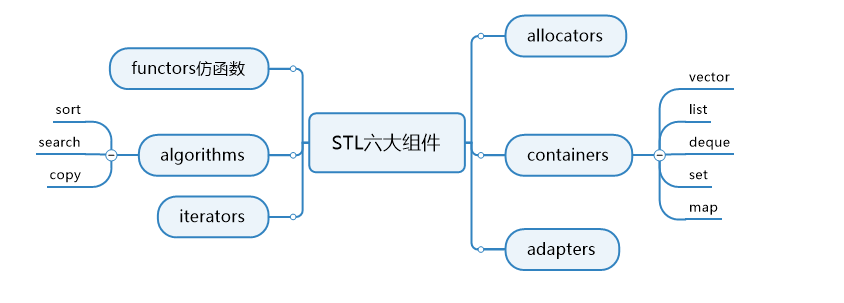
image-20240323151905853

Container通过Allocator取得数据储存空间，Algorithm通过Iterator存取Container内容，Functor可以协助Algorithm完成不同的策略变化，Adapter可以修饰或者套接Functor。

关联式容器associative containers

Set

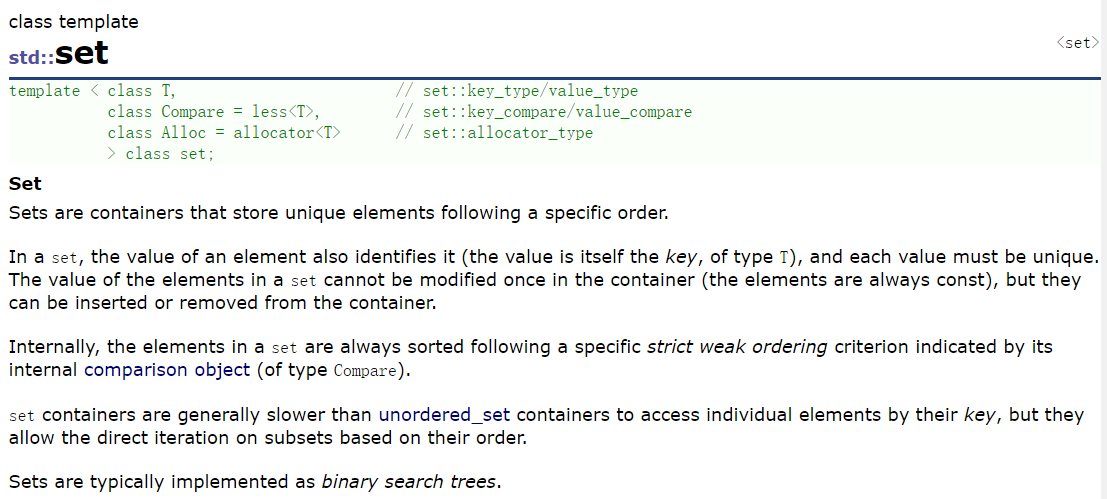
image-20240428231352539

  1. set是按照一定次序存储元素的容器
  2. 在set中，元素的value也标识它(value就是key，类型为T)，并且每个value必须是唯一的。set中的元素不能在容器中修改(元素总是const)，但是可以从容器中插入或删除它们。
  3. 在内部，set中的元素总是按照其内部比较对象(类型比较)所指示的特定严格弱排序准则进行排序。
  4. set容器通过key访问单个元素的速度通常比unordered_set容器慢，但它们允许根据顺序对子集进行直接迭代。
  5. set在底层是用二叉搜索树(红黑树)实现的

注意：

  1. 与map/multimap不同，map/multimap中存储的是真正的键值对<key, value>，set中只放value，但在底层实际存放的是由<value, value>构成的键值对。

  2. set中的元素不可以重复(因此可以使用set进行去重)。multiset能插入重复的值，如果查找的话找的第一个节点是中序遍历的第一个节点

  3. set中的元素默认按照小于来比较，使用set的迭代器遍历set中的元素，可以得到有序序列

set的基本使用

//构造
void const()
{std::set<int> first;                           // empty set of intsint myints[]= {10,20,30,40,50};std::set<int> second (myints,myints+5);        // rangestd::set<int> third (second);                  // a copy of secondstd::set<int> fourth (second.begin(), second.end());  // iterator ctor.std::set<int,classcomp> fifth;                 // class as Compare
}//插入
void insert()
{std::set<int> myset;std::set<int>::iterator it;std::pair<std::set<int>::iterator, bool> ret;for(int i = 1; i <= 5; i++) myset.insert(i);ret = myset.insert(2); //插入失败if(ret.second == false) it = ret.first; //指向20myset.insert(it, 9);for(it = myset.begin(); it != myset.end(); it++){cout << *it <<" ";}
}//查找
void find()
{std::set<int> myset;std::set<int>::iterator it;for(int i = 1; i <= 5; i++) myset.insert(i);it = myset.find(3);myset.erase(it);
}

Map

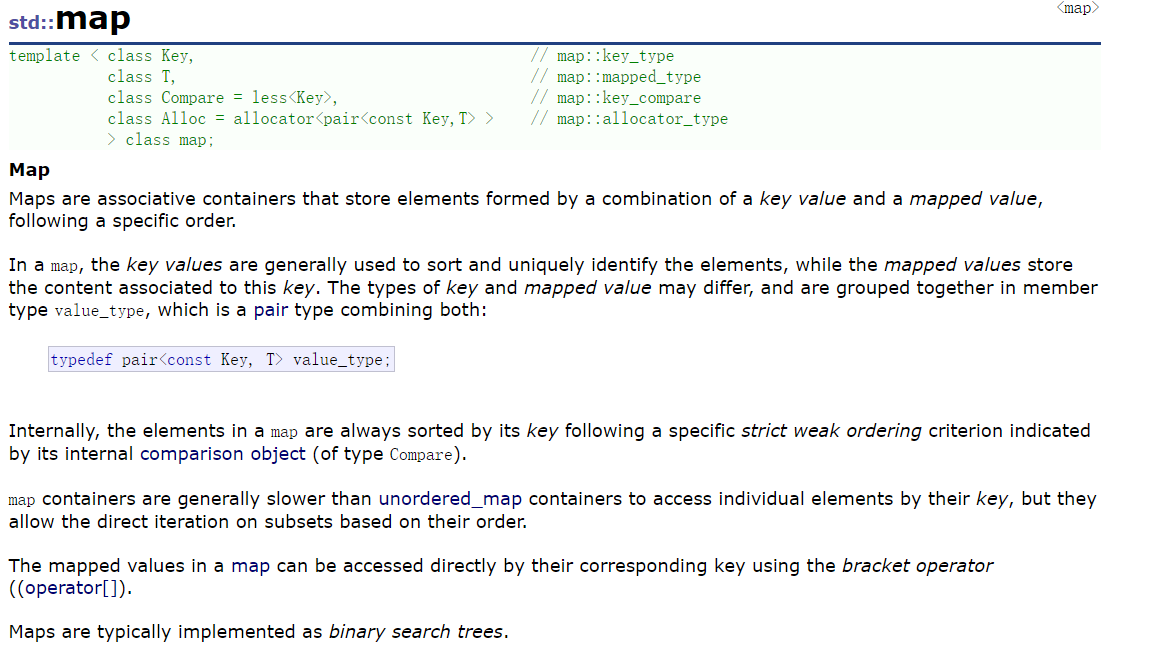
image-20240428233232502

  1. map是关联容器，它按照特定的次序(按照key来比较)存储由键值key和值value组合而成的元素,key值是不能修改的

  2. 在map中，键值key通常用于排序和惟一地标识元素，而值value中存储与此键值key关联的内容。键值key和值value的类型可能不同，并且在map的内部，key与value通过成员类型value_type绑定在一起，为其取别名称为pair

  3. 在内部，map中的元素总是按照键值key进行比较排序的。

  4. map中通过键值访问单个元素的速度通常比unordered_map容器慢，但map允许根据顺序对元素进行直接迭代(即对map中的元素进行迭代时，可以得到一个有序的序列)。

  5. map支持下标访问符，即在[]中放入key，就可以找到与key对应的value。

  6. map通常被实现为二叉搜索树(更准确的说：平衡二叉搜索树(红黑树))。

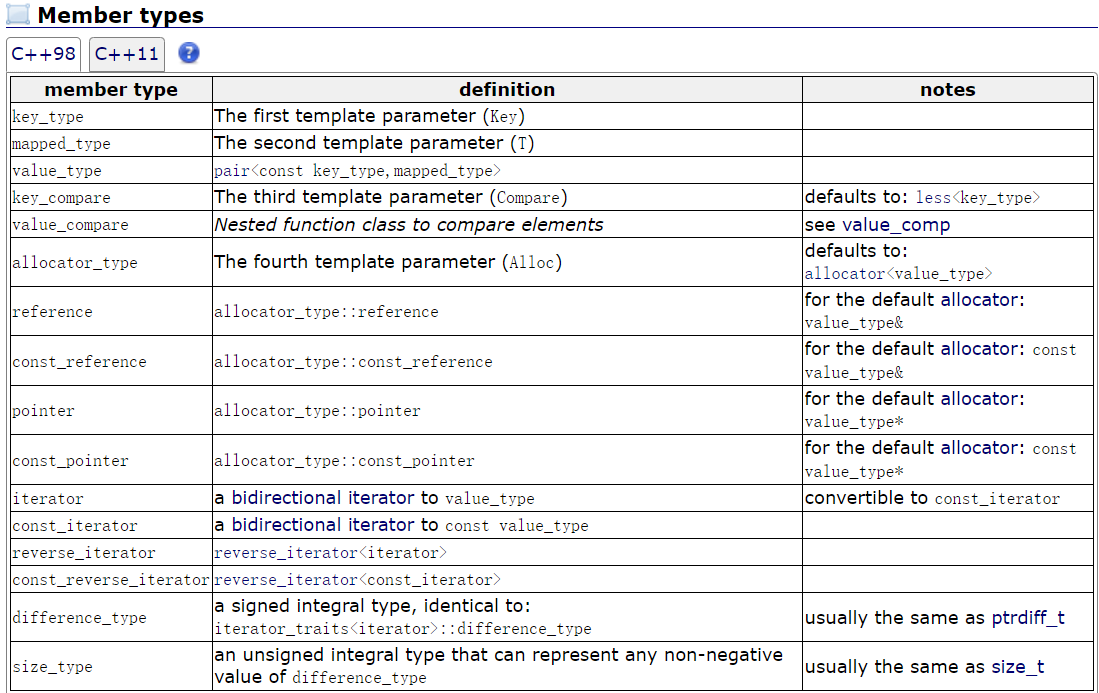
    image-20240428233831725

在正式使用Map之前先看看pair和make_pair

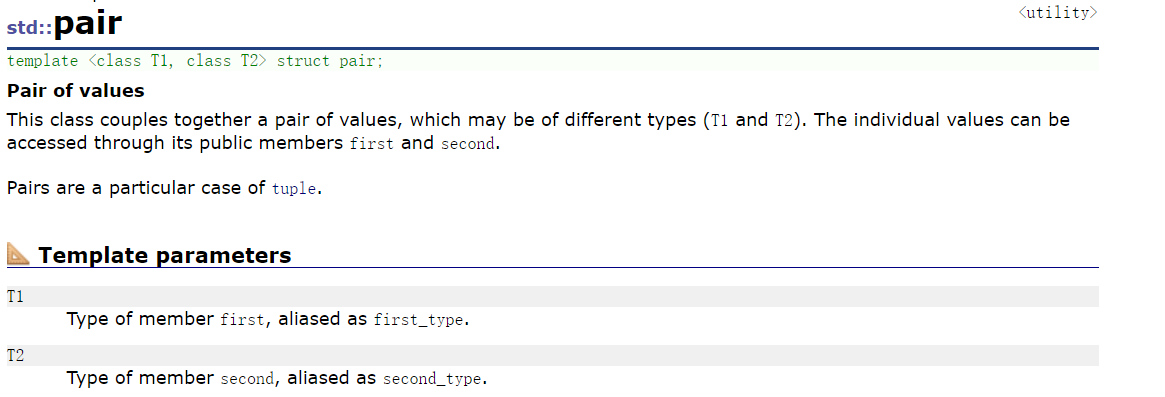
image-20240428233452384

make_pair在pair的基础上做了一个自动推导

image-20240428234502828

可以看到pair是一个结构体里面有2个值，第一个叫first，第二个值叫second。在map中first的值就是key，second的值是value。

Map的使用

//pair<iterator,bool> insert (const value_type& val);
//返回值是一个pair
void  insert()
{map<string, string> dict;//假设插入失败auto it = dict.insert(make_pair("peach","桃子"));cout<< it.first->second <<end; //false
}
int main()
{pair<string, string> kv("apple", "苹果");map<string, string> dict;dict.insert(kv);   dict.insert(pair<string, string>("banana", "香蕉"); //匿名对象dict.inset(make_pair("grape", "葡萄"));map<string, string>::iterator it = dict.begin();while(it != dict.end()){// cout << *(it).first << ":" << *(it).second << " ";cout << it->first << ":" << it->second <<" " ; // map里面->进行了重载 ->->}
}

map中的[]

在C++中，可以通过下标操作符[]来访问和修改Map中的值。如果要修改一个键对应的值，可以直接用下标操作符访问该键然后赋予新值。如果该键不存在，则会在Map中创建一个新键，并赋予默认初始化的值。

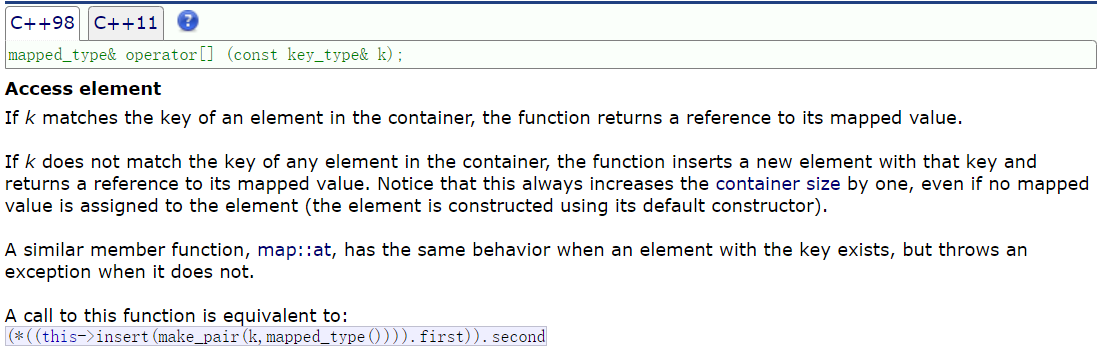
image-20240506200301855

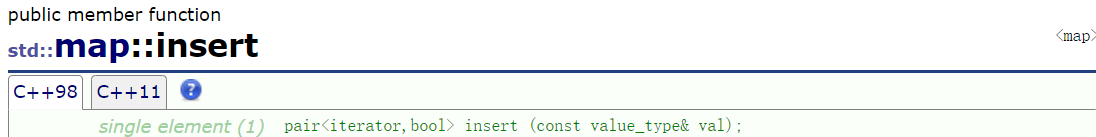
image-20240506200623063

调用完inset返回的是一个pair，.first取出iterator，iterator是指向插入的或者是已近存在的那个元素，*(iterator).first得到pair<const Key, T>，再.second得到T。

红黑树

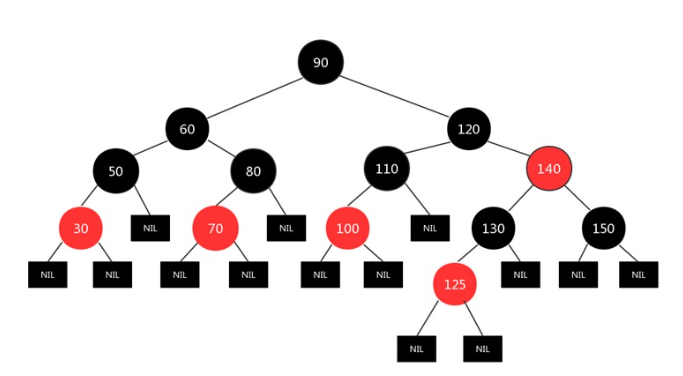
image-20240506195546485

红黑树的性质：

  1. 每个节点要么是红色，要么是黑色。
  2. 根节点是黑色。
  3. 每个叶子节点（NIL节点或空节点）是黑色。注意，这里的叶子节点指的是NIL节点或空节点。(NIL节点在红黑树中通常指的是空节点或叶子节点的占位符。在红黑树的实现中，为了简化逻辑和保证性质，通常假设每个节点都有两个子节点，即使这些子节点实际上并不存在。这些不存在的子节点由NIL节点（或称为空节点、哑节点、哨兵节点）来表示。)
  4. 如果一个节点是红色的，则它的两个子节点都是黑色的。这一性质保证了不会出现连续的红色节点，有助于维护树的平衡性。
  5. 从任一节点到其每个叶子节点的所有路径都包含相同数目的黑色节点。这一性质被称为“黑色节点路径长度相等”，它确保了红黑树的高度始终在对数级别。

模拟实现红黑树

红黑树节点

enum Color{RED,BLACK};template<class K, class V>struct RBTreeNode{RBTreeNode<K, V>* _left;RBTreeNode<K, V>* _parent;RBTreeNode<K, V>* _right;Color _col;pair<K, V> _kv;RBTreeNode(const pair<K, V>& kv):_left(nullptr), _parent(nullptr), _right(nullptr), _col(RED) //默认给红色, _kv(kv){}};

红黑树

//插入
bool insert(const pair<K, V>& kv)
{if (_root == nullptr){_root = new Node(kv);_root->_col = BLACK;return true;}Node* cur = _root;Node* parent = nullptr;while (cur){if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else{return false;}}cur = new Node(kv);cur->_col = RED;if (parent->_kv.first > kv.first){parent->_left = cur;cur->_parent = parent;}else{parent->_right = cur;cur->_parent = parent;}//控制平衡while (parent && parent->_col == RED){Node* g = parent->_parent;if (parent == g->_left){Node* u = g->_right;//1.u存在且为红if (u && u->_col == RED){//变色parent->_col = BLACK;u->_col = BLACK;g->_col = RED;// 向上处理cur = g;parent = cur->_parent;}//u不存在/u存在且为黑else{//          g//       p//  cur//右单旋if (cur == parent->_left){RotateR(g);g->_col = RED;parent->_col = BLACK;}//      g//   p//      cur//左右双旋else{RotateL(parent);RotateR(g);cur->_col = BLACK;g->_col = RED;}break;}}//(parent == g->_right)else{Node* u = g->_left;//1.u存在且为红if (u&& u->_col == RED){//变色parent->_col = BLACK;u->_col = BLACK;g->_col = RED;// 向上处理cur = g;parent = cur->_parent;}//u不存在 /u存在且为黑else{//    g//       p//          curif (parent->_right = cur){RotateL(g);g->_col = RED;parent->_col = BLACK;}//    g//        p//   curelse{RotateR(parent);RotateL(g);cur->_col = BLACK;g->_col = RED;}break;}}}_root->_col = BLACK;return true;
}//判断平衡bool _IsBalance(Node* root, const int refernum, int actualnum)
{if (root == nullptr){if (refernum != actualnum){cout << "实际值的黑色节点数目和参考值不一样" << endl;return false;}else return true;}if (root->_col == RED && root->_parent->_col == RED){cout << "parent和cur都为红，出现连续的红节点" << endl;return false;}if (root->_col == BLACK)++actualnum;return _IsBalance(root->_left, refernum, actualnum)&& _IsBalance(root->_right, refernum, actualnum);}
bool IsBalance()
{if (_root && _root->_col == RED){cout << "根节点为红色" << endl;return false;}//选择一条路径记录黑色节点的数量做参考值int refernum = 0;Node* left = _root;while (left){if(left->_col == BLACK){refernum++;left = left->_left;}}int actualnum = 0;return _IsBalance(_root, refernum, actualnum);
}

红黑树模拟实现STL中的map和set

由于想要红黑树即能存k也能存pair，所以对上面的代码进行改造

红黑树部分

enum Color
{RED,BLACK
};//T 代表  K或者是 pair<K,V>
template<class T>
struct RBTreeNode
{RBTreeNode<T>* _left;RBTreeNode<T>* _parent;RBTreeNode<T>* _right;Color _col;T _date; //用date,因为值是什么类型的并不知道RBTreeNode(const T& date):_left(nullptr), _parent(nullptr), _right(nullptr), _col(RED) //默认给红色, _date(date){}
};//map <class k,class V,MapKeyOfT>
//set <class k,class k,SetKeyOfT>
//通过KeyOfT返回值来用 
template < class K, class T, class KeyOfT>
class RBTree
{
public:typedef RBTreeNode<T> Node;typedef RBTreeIterator<T, T&, T*> iterator;typedef RBTreeIterator<T, const T&, const T*> const_iterator;//开始就是中序最小的值iterator begin() {Node* cur = _root;while (cur&& cur->_left)cur = cur->_left;return iterator(cur);}iterator end(){return iterator(nullptr);}RBTree():_root(nullptr){}RBTree(const RBTree<K, T, KeyOfT>& tree){_root = Copy(tree._root);}RBTree<K, T, KeyOfT>& operator=(RBTree<K, T, KeyOfT> t){swap(_root, t);return *this;}~RBTree(){Destroy(_root);_root = nullptr;}
private:void Destroy(Node* root){if (root == nullptr){return;}Destroy(root->_left);Destroy(root->_right);delete root;}Node * Copy(Node* root){if (root == nullptr)return nullptr;//生成一个新根+换颜色Node* newtree = new Node(root->_date);newtree->_col = root->_col;newtree->_left = Copy(root->_left);newtree->_right = Copy(root->_right);//处理newtree的parentif (newtree->_left)newtree->_left->_parent = newtree;else{newtree->_right->_parent = newtree;			}return newtree; }void RotateR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;Node* parentparent = parent->_parent;subL->_right = parent;parent->_parent = subL;if (parent == _root){_root = subL;_root->_parent = nullptr;}//parent是别人的子树else{if (parentparent->_left == parent)parentparent->_left = subL;elseparentparent->_right = subL;subL->_parent = parentparent;}parent->_left = subLR;//subLR 可能不存在if (subLR){subLR->_parent = parent;}}void RotateL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;if (subRL){subRL->_parent = parent;}Node* parentparent = parent->_parent;if (parent == _root){_root = subR;subR->_parent = nullptr;}else{if (parentparent->_left == parent)parentparent->_left = subR;elseparentparent->_right = subR;subR->_parent = parentparent;}subR->_left = parent;parent->_parent = subR;parent->_right = subRL;}public:pair<iterator, bool> insert(const T& date){if (_root == nullptr){_root = new Node(date);_root->_col = BLACK;return make_pair(iterator(_root),true);}Node* cur = _root;Node* parent = nullptr;KeyOfT key;while (cur){if (key(cur->_date) > key(date)){parent = cur;cur = cur->_left;}else if (key(cur->_date) < key(date)){parent = cur;cur = cur->_right;}else{return make_pair(iterator(cur), false);}}cur = new Node(date);Node* newnode = cur;cur->_col = RED;//新增给红,只影响新增所在的路径if (key(parent->_date) > key(date)){parent->_left = cur;cur->_parent = parent;}else{parent->_right = cur;cur->_parent = parent;}//控制平衡while (parent && parent->_col == RED){Node* g = parent->_parent;if (parent == g->_left){Node*u = g->_right;//1.u存在且为红if (u&& u->_col == RED){//变色parent->_col = BLACK;u->_col = BLACK;g->_col = RED;// 向上处理cur = g;parent = cur->_parent;}//u不存在/u存在且为黑else{//          g//       p//  cur//右单旋if (cur == parent->_left){RotateR(g);g->_col = RED;parent->_col = BLACK;}//      g//   p//      cur//左右双旋else{RotateL(parent);RotateR(g);cur->_col = BLACK;g->_col = RED;}break;}}//(parent == g->_right)else{Node* u = g->_left;//1.u存在且为红if (u&& u->_col == RED){//变色parent->_col = BLACK;u->_col = BLACK;g->_col = RED;// 向上处理cur = g;parent = cur->_parent;}//u不存在 /u存在且为黑else{//    g//       p//          curif (parent->_right = cur){RotateL(g);g->_col = RED;parent->_col = BLACK;}//    g//        p//   curelse{RotateR(parent);RotateL(g);cur->_col = BLACK;g->_col = RED;}break;}}}_root->_col = BLACK;return make_pair(iterator(newnode), true);}private:Node* _root;};

红黑树迭代器

template<class T, class Ref, class Ptr>
struct RBTreeIterator
{typedef RBTreeNode<T> Node;typedef RBTreeIterator<T, Ref, Ptr>Self;Node* _node;//节点来构造迭代器RBTreeIterator(Node* node):_node(node){}Ref operator*(){return _node->_date;}Ptr operator->(){return &_node->_date;//返回date的地址 ->优先级高}//中序Self& operator++(){// 1.如果右树不为空就访问右树最左节点if (_node->_right){Node* min = _node->_right;while (min->_left){min = min->_left;}_node = min;}//2.右树为空，说明所在子树访问完了else{Node* cur = _node;Node* parent = cur->_parent;//沿着到根路径往上走while (parent && cur == parent->_right){cur = cur->_parent;parent = parent->_parent;}//找左孩子是父亲的祖父节点，继续更新直到父亲为空_node = parent;}return *this;}Self& operator--(){//1.访问左子树最右节点if (_node->_left){Node* max= _node->_left;while (max->_right){max = max->_right;}_node = max;}//2.左为空说明访问完了else{Node* cur = _node;Node* parent = cur->_parent;//沿着到根路径往上走while (parent &&cur==parent->left){cur = cur->_parent;parent = parent->_parent;}//找右孩子是父亲的祖父节点，继续更新直到父亲为空_node = parent;}return *this;}bool operator!=(const Self& s) const{return _node != s._node;}bool operator==(const Self& s)  const{return _node == s._node;}};

map.h

#pragma once
namespace jt
{template<class K,class V>class map{public:struct MapKeyOfT{const K& operator()(const pair<K,V>& kv){return kv.first;}};typedef typename RBTree<K, pair<K, V>, MapKeyOfT>::iterator iterator;iterator begin(){return _t.begin();}iterator end(){return _t.end();}pair<iterator, bool> insert(const pair<K, V>& kv){return _t.insert(kv);}V& operator[](const K& key){auto ret = _t.insert(make_pair(key, V()));return ret.first->second;}private:RBTree<K, pair<K, V>, MapKeyOfT> _t;};}

set.h

namespace jt
{template<class K>class set{public:struct SetKeyOfT{const K& operator()(const K& k){return k;}};typedef typename RBTree<K, K, SetKeyOfT>::iterator iterator;iterator begin(){return _t.begin();}iterator end(){return _t.end();}pair<iterator, bool> insert(const K& key){return _t.insert(key);}private:RBTree<K, K, SetKeyOfT> _t;};}

map和set是适配器吗？

容器：容器是存储数据的对象，这些对象提供了对它们所包含元素的访问。C++ 标准库提供了多种容器，如 vector, list, deque, map, set, unordered_map, unordered_set 等。

适配器：适配器是设计模式中的一种，它允许将一个类的接口转换为客户端所期望的另一个接口。在 C++ 标准库中，适配器通常是指那些不直接存储数据，但提供对数据的访问或修改操作的类。常见的适配器包括 stack, queue, priority_queue 等，它们通常基于其他容器（如 deque）来实现。

map 和 set 是直接存储数据的容器。它们内部有自己的数据结构（通常是红黑树）来存储和管理元素。与此不同，适配器通常不直接存储数据，而是基于其他容器来提供特定的接口或行为。

例如，stack 是一个适配器，它基于一个底层容器（如 deque）来提供栈的行为（如 push, pop, top 等）。但 stack 本身并不存储数据，它只是将 deque 的接口转换为栈的接口。

另一方面，map 提供了键值对的存储和查找，而 set 提供了唯一元素的存储和查找。这些功能是通过它们自己的数据结构和算法来实现的，而不是通过适配其他容器的接口。因此，map 和 set 被归类为容器，而不是适配器。

红黑树与AVL树对比

红黑树和AVL树都是高效的平衡二叉树，增删改查的时间复杂度都是O(log2N)，红黑树不追求绝对平衡，其只需保证最长路径不超过最短路径的2倍，相对而言，降低了插入和旋转的次数，红黑树的设计确保了任何不平衡都可以在三次旋转之内解决。所以在经常进行增删的结构中性能比AVL树更优，而且红黑树实现比较简单，所以实际运用中红黑树更多。

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://xiahunao.cn/news/3031801.html

如若内容造成侵权/违法违规/事实不符，请联系瞎胡闹网进行投诉反馈，一经查实，立即删除！

相关文章

【XR806开发板试用】试用SWD+Jlink调试

【XR806开发板试用】试用SWD+Jlink调试

XR806开发板&#xff0c;只能使用编写代码&#xff0c;然后通过UART下载&#xff0c;没法在线debug&#xff0c; 效率会差很多&#xff0c;官方没有提供这一方面的资料。 先查CPU&#xff0c; 官方介绍是arm-china的MC1&#xff0c;通过armv8 Architecture refenence manual资料…
阅读更多...
利用106短信群发平台能否提升沟通效率？

利用106短信群发平台能否提升沟通效率？

利用106短信群发平台确实能够显著提升沟通效率&#xff0c;具体体现在以下几个方面&#xff1a; 1.快速传递信息&#xff1a;106短信群发平台能够实现信息的快速传递。一旦设置好发送内容和接收群体&#xff0c;短信便能在瞬间发送至大量用户。这种即时性确保了信息的迅速传达…
阅读更多...
Gitlab：从其它项目组里导入一个项目

Gitlab：从其它项目组里导入一个项目

1.首先获取原项目的http地址 http://ip/projectGroup/ProjectX.git其中&#xff0c;ip 为公司gitlab内网地址。 2.进入目的项目组进行创建 首先&#xff0c;需要拥有一个该组拥有者权限的账号&#xff0c;才能进行后续的操作。 2.1.点击创建项目按钮 2.2.选择导入项目 其中…
阅读更多...
11.买卖股票的最佳时机Ⅰ

11.买卖股票的最佳时机Ⅰ

文章目录 题目简介题目解答解法一&#xff1a;一次遍历代码&#xff1a;复杂度分析&#xff1a; 题目链接 大家好&#xff0c;我是晓星航。今天为大家带来的是 买卖股票的最佳时机面试题Ⅰ 相关的讲解&#xff01;&#x1f600; 题目简介 题目解答 解法一&#xff1a;一次遍历…
阅读更多...
怎么让电脑耳机和音响都有声音

怎么让电脑耳机和音响都有声音

电脑耳机音响不能同时用没声音怎么办 一般来说&#xff0c;重新开机后问题能够得到解决。右击“我的电脑”---“属性”---“硬件”---“设备管理器”&#xff0c;打开“声音、视频和游戏控制器”有无问题&#xff0c;即看前面有没有出现黄色的“”。 如果您的 电脑 耳机能正常…
阅读更多...
【Linux系统编程】第十六弹---冯诺依曼体系结构与操作系统

【Linux系统编程】第十六弹---冯诺依曼体系结构与操作系统

✨个人主页&#xff1a; 熬夜学编程的小林 &#x1f497;系列专栏&#xff1a; 【C语言详解】 【数据结构详解】【C详解】【Linux系统编程】 目录 1、冯诺依曼体系结构 2、操作系统原理 2.1、什么是操作系统&#xff1f; 2.2、用图解释操作系统 2.3、理解操作系统 总结 …
阅读更多...
路由器、交换机和网卡

路由器、交换机和网卡

大家使用VMware安装镜像之后&#xff0c;是不是都会考虑虚拟机的镜像系统怎么连上网的&#xff0c;它的连接方式是什么&#xff0c;它ip是什么&#xff1f; 路由器、交换机和网卡 1.路由器 一般有几个功能&#xff0c;第一个是网关、第二个是扩展有线网络端口、第三个是WiFi功…
阅读更多...
在做题中学习（55）：一维前缀和模板

在做题中学习（55）：一维前缀和模板

【模板】前缀和_牛客题霸_牛客网 (nowcoder.com) 题目解释&#xff1a; 注意&#xff1a;下标从1开始的。 l 和 r就是对这n个整数去取一个区间&#xff0c;例如示例一&#xff1a; (1,2) 区间 就是算出1 2 4 中 1&#xff0c;2下标对应值的和&#xff0c;12 3 同理,(2,3) …
阅读更多...
SHAP分析+立方样条拟合的展示可能的交互作用

SHAP分析+立方样条拟合的展示可能的交互作用

SHAP分析立方样条的拟合展示可能的交互作用 SHAP分析的另一个特点就是对交互作用的分析&#xff0c;计算交互作用的SHAP值&#xff0c;绘制相关的交互作用图表&#xff0c;但是仅局限于xgboost模型&#xff0c;其它的模型不能单独计算相互作用的SHAP值&#xff0c;也就不能绘制…
阅读更多...
React 第二十九章 React 和 Vue 描述页面的区别

React 第二十九章 React 和 Vue 描述页面的区别

面试题&#xff1a;React 和 Vue 是如何描述 UI 界面的&#xff1f;有一些什么样的区别&#xff1f; 标准且浅显的回答&#xff1a; React 中使用的是 JSX&#xff0c;Vue 中使用的是模板来描述界面 前端领域经过长期的发展&#xff0c;目前有两种主流的描述 UI 的方案&#xf…
阅读更多...
读写备份寄存器BKP与实时时钟RTC

读写备份寄存器BKP与实时时钟RTC

文章目录 读写备份寄存器接线图代码 RTC实时时钟接线图代码 读写备份寄存器 接线图 即接个3.3v的电源到VBT引脚 代码 代码效果&#xff1a;第一次写入备份寄存器&#xff0c;下载程序后再注释掉&#xff0c;再进行下载&#xff0c;之前写入的数据还会保存在备份寄存器中&am…
阅读更多...
QQ无人直播秘籍：24小时自动化短剧，边睡边赚，月入过万实战分享！

QQ无人直播秘籍：24小时自动化短剧，边睡边赚，月入过万实战分享！

在如今经济大环境如此糟糕的时代&#xff0c;寻找一种简单、有效的方式来实现日入1000的梦想成为了许多人的追求。而QQ短剧24小时无人直播项目&#xff0c;则是一个备受瞩目的赚钱机会。借助腾讯官方流量扶持&#xff0c;这个项目不仅能够让创业者轻松入门&#xff0c;还能够实…
阅读更多...
新火种AI|正面硬刚OpenAI与谷歌？微软竟然偷偷自研出5000亿参数大模型！

新火种AI|正面硬刚OpenAI与谷歌？微软竟然偷偷自研出5000亿参数大模型！

在AI领域&#xff0c;微软公司一直以其独到的创新性和前瞻性而闻名。也正因此&#xff0c;它抢先在AI赛道嗅到商机&#xff0c;并极具预判性的投资了OpenAI&#xff0c;使其成为自己在AI赛道上的最强助力。不过&#xff0c;微软的野心不止于此。 根据The Information 5月6日的…
阅读更多...
光耦推荐—高速风筒方案中用到哪些光耦型号

光耦推荐—高速风筒方案中用到哪些光耦型号

高速风筒是现代生活中常见的电器设备&#xff0c;广泛应用于家庭、商业和工业领域&#xff1b;光耦是一种能够将输入信号转换成输出信号的元器件&#xff0c;其作用在于将电气信号转换成光信号&#xff0c;从而实现电路的隔离和保护&#xff1b;采用光耦可实现对风机转速和温度…
阅读更多...
品牌控价前要先了解目标

品牌控价前要先了解目标

对渠道中出现的低价链接、窜货链接进行治理的过程&#xff0c;就叫做控价&#xff0c;控价的最终目的是为使这些低价链接下架或者改价&#xff0c;但不是所有品牌都只做打击&#xff0c;便能管控好渠道的&#xff0c;也就是说&#xff0c;控价的方法不能只依靠治理&#xff0c;…
阅读更多...
【CTFHub】HTTP 请求方式 302跳转 cookie WP

【CTFHub】HTTP 请求方式 302跳转 cookie WP

1.请求方式 打开给出的URL进入一个页面&#xff0c;提示原方法是GET&#xff0c;用CTFHUB方法就能获得flag 思路&#xff1a;抓包&#xff0c;将GET方法改成CTFHUB方法进行重新发送请求&#xff0c;查看响应情况 1.打开代理服务器 2.打开BurpSuite 刷新页面获得拦截 3.发送…
阅读更多...
【Centos7 】Centos7yum报错:another app is currently holding the yum lock；解决方案

【Centos7 】Centos7yum报错:another app is currently holding the yum lock；解决方案

Centos7 yum报错:another app is currently holding the yum lock;waiting for it to exit 大家好 我是寸铁&#x1f44a; 总结了一篇Centos7 yum报错:another app is currently holding the yum lock;waiting for it to exit✨ 喜欢的小伙伴可以点点关注 &#x1f49d; 报错 解…
阅读更多...
【UnityRPG游戏制作】Unity_RPG项目_玩法相关※

【UnityRPG游戏制作】Unity_RPG项目_玩法相关※

&#x1f468;‍&#x1f4bb;个人主页&#xff1a;元宇宙-秩沅 &#x1f468;‍&#x1f4bb; hallo 欢迎 点赞&#x1f44d; 收藏⭐ 留言&#x1f4dd; 加关注✅! &#x1f468;‍&#x1f4bb; 本文由 秩沅 原创 &#x1f468;‍&#x1f4bb; 收录于专栏&#xff1a;就业…
阅读更多...
认识下MapReduce

认识下MapReduce

&#x1f50d; 什么是MapReduce&#xff1f; MapReduce是一种分布式计算模型&#xff0c;最初由Google提出&#xff0c;用于处理大规模数据集的并行计算。它将数据处理任务分解成独立的Map和Reduce两个阶段&#xff0c;以实现分布式计算和并行化处理。Map阶段负责将输入数据映…
阅读更多...
Web3Tools - 助记词生成

Web3Tools - 助记词生成

Web3Tools - 助记词生成工具 本文介绍了一个简单的助记词生成工具&#xff0c;使用 React 和 Material-UI 构建。用户可以选择助记词的语言和长度&#xff0c;然后生成随机的助记词并显示在页面上 功能介绍 选择语言和长度&#xff1a; 用户可以在下拉菜单中选择助记词的语言&…
阅读更多...
最新文章

Copyright @ 2022~2023