Taxi Cab Scheme
题意
有一张边长不超过 200 200 200 的网格图,有若干个乘客,
乘客 i i i 的需求是: h h : m m , ( a , b ) , ( c , d ) hh:mm, (a,b) , (c, d) hh:mm,(a,b),(c,d),意为他需要在 h h 时 m m 分 hh时mm分 hh时mm分(或更早)从点 ( a , b ) (a,b) (a,b) 出发,终点为 ( c , d ) (c,d) (c,d)
图上两点的距离就是 曼哈顿距离
现在我们需要使用一些出租车来满足这些乘客的需求,问最少需要几台车?
一台车必须在当前要接送的顾客要求的时间更早到达起点(起码 1 m i n 1min 1min),才能接送成功
思路
最优的情况是只用一台车,那么这台车每次接完当前顾客,它都需要从这个顾客的终点移动到下一个顾客的起点,并且最终到达的时间必须比下一个顾客的要求时间早
那么我们不妨先预处理一下每个乘客的结束时间,存储一下起始时间,并将所有时间转换成 分钟制,便于比较
如果送完了当前顾客 i i i,还能接送下一个顾客 j j j,那么我们可以连边: i → j i \rarr j i→j,表示这个传递关系
那么我们 O ( n 2 ) O(n^2) O(n2) 连完边后,从结果出发,如果我们选择了若干辆出租车,每辆出租车按一条路径这样搜索下去,直到不能搜素为止,最终每一个点都被访问了一次
可以发现:关键就是怎么选取这个起始点?也就是选择哪些点作为第一批送走的顾客
我们再进一步想:如果我们选择的集合里面,有两个点,其中一个点可以被另一个点搜索到,也就是说这个点是多余的,那么这个点可以删去
那么我们可以得出结论:最终选择的点集,一定是两两不可达的,等价于集合内没有边,
等价于集合是独立集
我们可以在这张二分图上跑最大匹配,那么最终残余网络上,右点还有容量的点就是起点,左点还有容量的点就是终点,由于已经跑出了最大流量,所以残余网络不会变得更小,因此这个起点集就是最小的
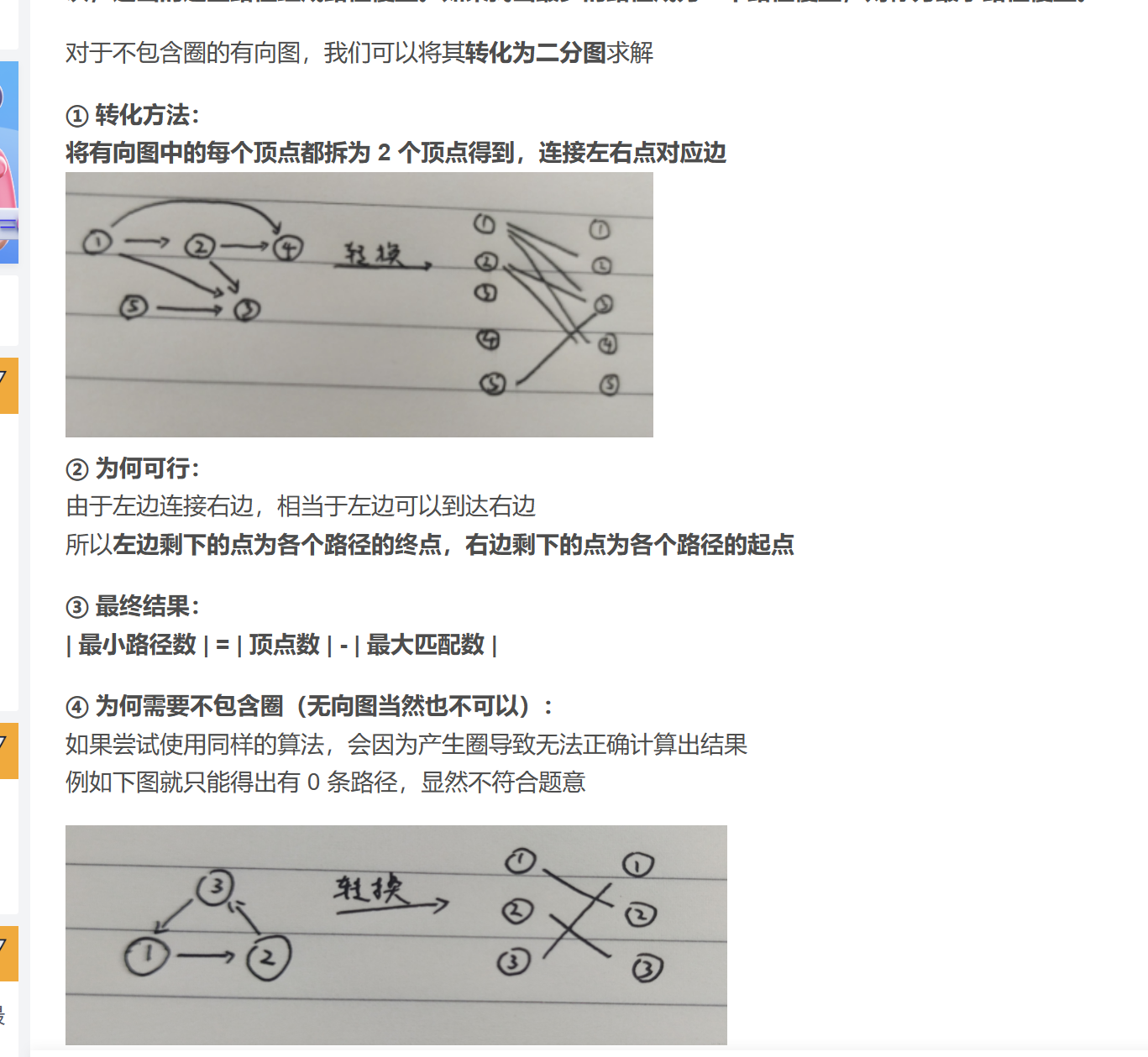
部分思路参考自:最小路径覆盖详解 超级详细
#include<bits/stdc++.h>
#define fore(i,l,r) for(int i=(int)(l);i<(int)(r);++i)
#define fi first
#define se second
#define endl '\n'
#define ull unsigned long long
#define ALL(v) v.begin(), v.end()
#define Debug(x, ed) std::cerr << #x << " = " << x << ed;const int INF=0x3f3f3f3f;
const long long INFLL=1e18;typedef long long ll;std::vector<std::vector<int>> g;
std::vector<int> match;
std::vector<bool> used;bool dfs(int u){for(auto v : g[u])if(!used[v]){used[v] = true;if(match[v] == -1 || dfs(match[v])){match[v] = u;return true;}}return false;
}int main(){std::ios::sync_with_stdio(false);std::cin.tie(nullptr);std::cout.tie(nullptr);int t;std::cin >> t;while(t--){int n;std::cin >> n;g.assign(n, std::vector<int>());match.assign(n, -1);std::vector<std::pair<int, int>> p(n); //source posstd::vector<std::pair<int, int>> pe(n); //end posstd::vector<std::pair<int, int>> time(n); //beg, endfore(i, 0, n){std::string s;std::cin >> s >> p[i].fi >> p[i].se;int x, y;std::cin >> x >> y;pe[i] = {x, y};int dis = std::abs(p[i].fi - x) + std::abs(p[i].se - y);int t0 = atoi(s.substr(0, 2).c_str()) * 60 + atoi(s.substr(3).c_str());time[i] = {t0, t0 + dis};} fore(i, 0, n)fore(j, i + 1, n){auto [x1, y1] = pe[i];auto [x2, y2] = p[j];int dis = std::abs(x1 - x2) + std::abs(y1 - y2);if(time[i].se + dis < time[j].fi){g[i].push_back(j);}}int cnt = 0;fore(i, 0, n){used.assign(n, false);cnt += dfs(i);}std::cout << n - cnt << endl;}return 0;
}